Molar absorptivity is the measure of how well an object absorbs radiation that the object is in contact with. Molar absorptivity is integral to Beer-Lambert law which relates absorbance, absorptivity, and concentration. Because of this relationship, rearranging the Beer-Lambert equation gives the formula for epsilon, or molar absorptivity below. The unit of measure for molar absorptivity is L mol-1 cm-1. In this article, you will learn to calculate absorptivity, and the practical applications.

The equation above shows an arrangement of Beer-Lambert law that solves for molar absorptivity. To better understand, absorptivity- the other components of the equation are crucial.
A in the equation above represents absorbance and is quantifiable by taking the log of incident (Io) and transmitted (I) intensity. The equation below relates the three terms to get a value of absorbance which is a unitless measure.
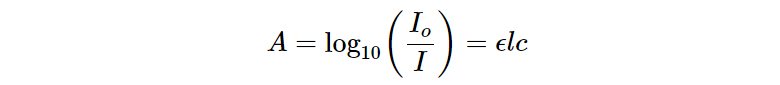
Absorbance refers to the amount of light or radiation an object is able to absorb. Additionally, absorptivity may also be referenced as optical density and is heavily dealt with in spectroscopy. Absorbance is directly proportional to the concentration and length (l) of a sample.
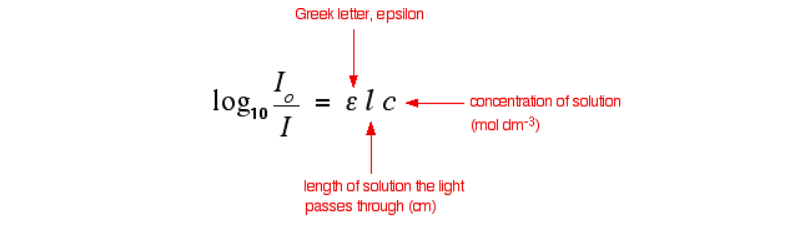
The l in the equation for absorptivity stands for length of the medium the light will pass through. The unit of measure must match that of c in the equation which is the concentration of solution. Additionally, the concentration of the solution is in units of mol/L. Concentration defines the ability of a solution or medium to absorb light. Therefore, the more concentration in a medium, the more molecules that are available to absorb light and other waves in a medium. The equation below relates and identifies these terms.
How to Calculate Molar Absorptivity
Using the equations above you may solve for absorptivity. The following sample calculation highlights the utilization of Beer’s law to calculate absorptivity.
Given Guanosine has an absorbance of .7 in a path of length 1cm, calculate the absorptivity given the concentration is about .0000833 mol/L.
In the same way, using Beer’s law we may easily fill in the formula that absorptivity is absorbance divided by the product of length and concentration to get the following:
Molar Absorptivity = (.7) /(1cm)(.0000833 mol/L) = 8433 M-1 cm-1
Practical Applications
Molar absorptivity is a crucial concept in a large variety of applications. In one major example, solar cells utilize concepts of absorptivity in order to excite cells from ground level to higher energy states in order to generate energy. Similarly, to identify the material type and it’s molar absorptivity is crucial in the output of high function and durable solar cells.
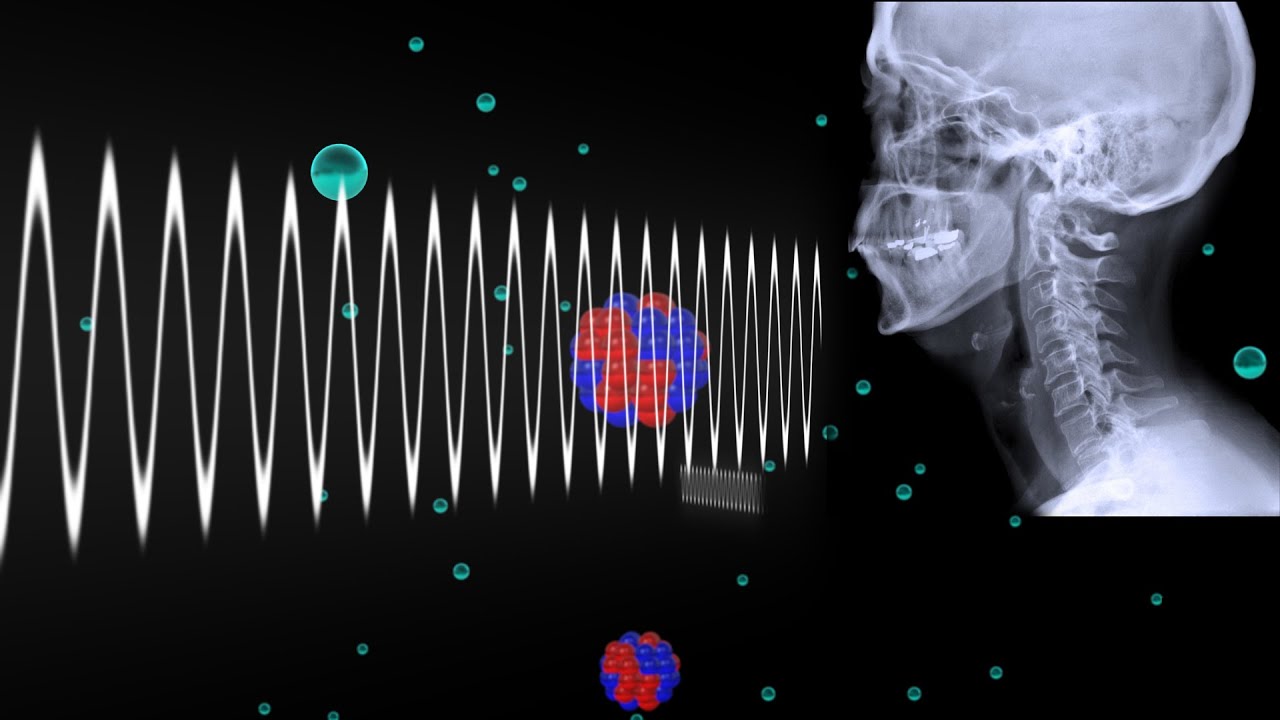
Additionally, another practical application for molar absorptivity is in the word of medical imaging and non-destructive examination. Both of these fields involve the use of x-rays, MRI, and other types of scans. The basic principle on which all of these methods work is that of absorptivity. Therefore, utilizing the right type of ray is important to imaging the internals of a human body or the grain structure of heterogeneous material. On the basis of molar absorptivity, rays go unabsorbed by the part being imaged but pass through parts not being imaged to create a scan of specified parts.



